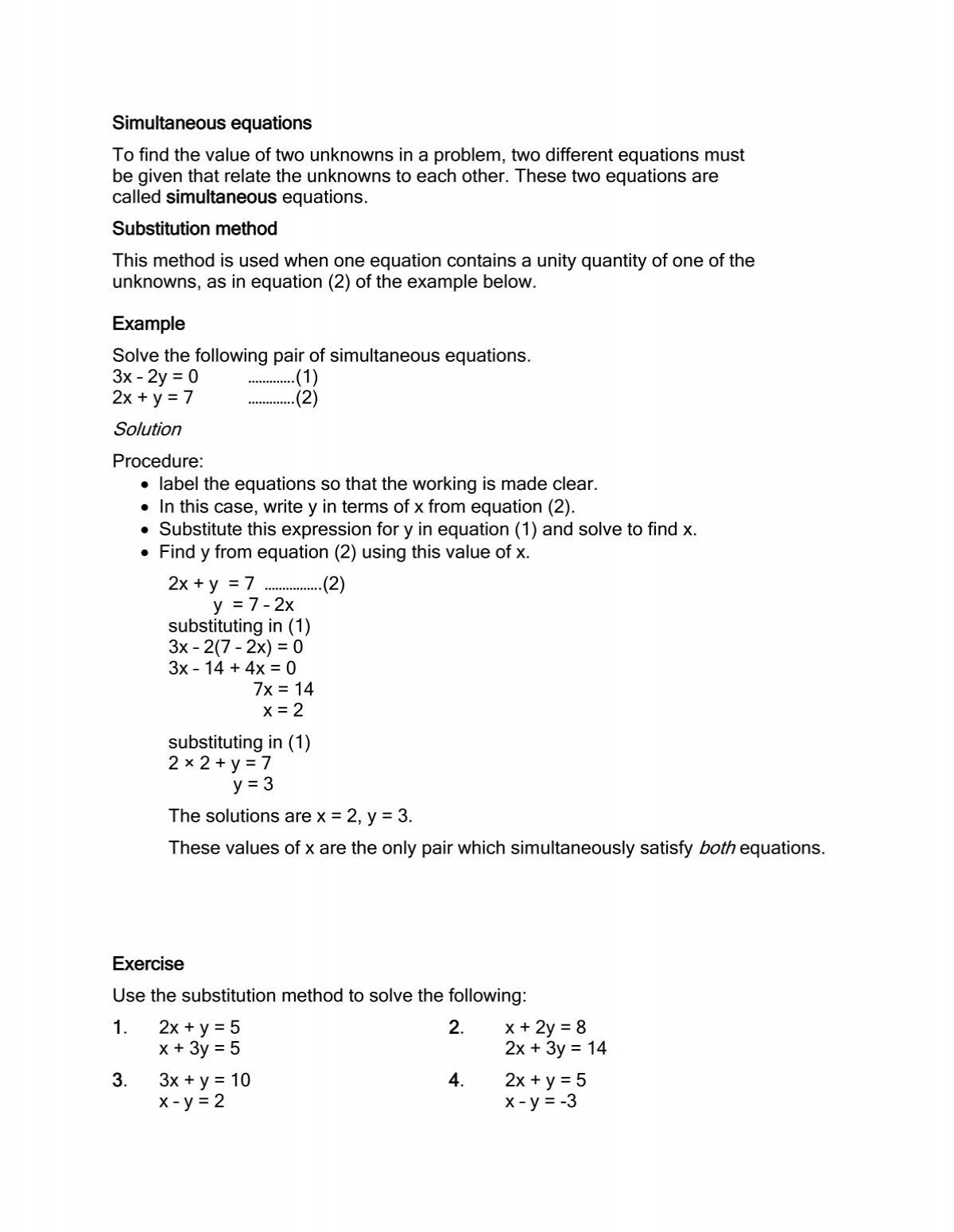
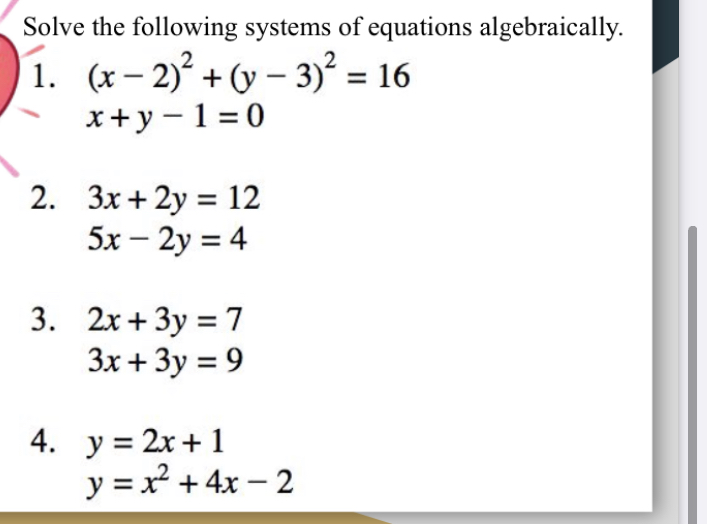
X 2y=3/2 2x Y=3/2 By Substitution Method 103313-X+2y=3/2 2x+y=3/2 By Substitution Method

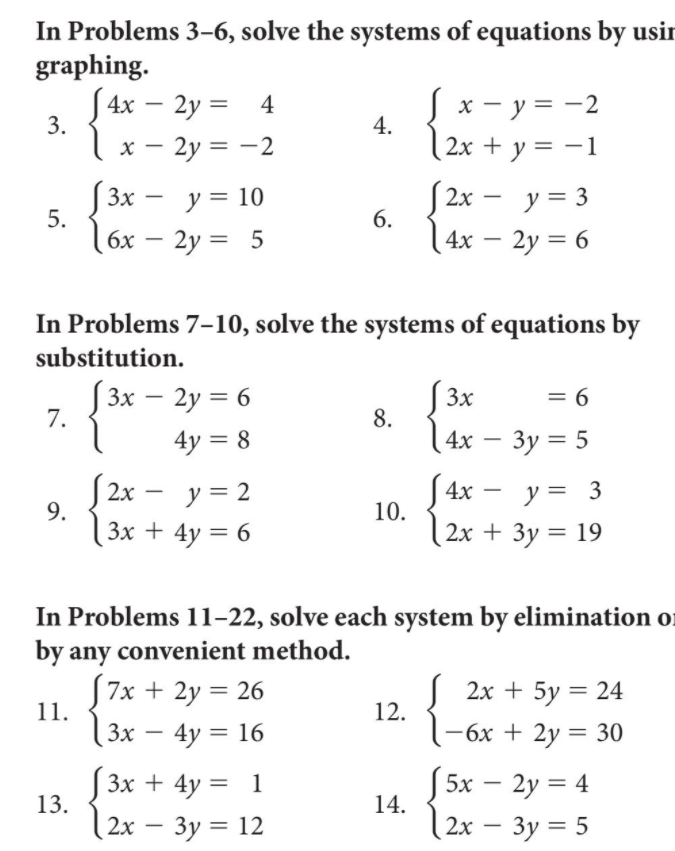
2xy=15,x2y=15 Pour calculer une paire d’équations à l’aide de la substitution, commencez par résoudre l’un des équations pour l’une des variables Substituez ensuite le résultat de cette variable dans l’autre équation Substituer 9 à y dans x2y=15 Comme l’équation résultante ne contient qu’une variable, vousCancel the common factor Divide y y by 1 1 Divide 3 3 by 3 3 Replace all occurrences of y y with 1 1 in each equation Tap for more steps Replace all occurrences of y y in x = y x = y with 1 1 Remove parentheses The solution to the system is the1 Solve using the substitution method y=2x3 y=x5 2 Solve using the elimination method 2xy=4 x2y = 3 3

2 2 Y 3 Store 53 Off Www Nogracias Org

X+2y=3/2 2x+y=3/2 by substitution method

X+2y=3/2 2x+y=3/2 by substitution method-Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Solve by substitution method x 2y = 1 and 2x 3y = 12 ⇒ x 2y = – 1 (i) ⇒ 2x – 3y = 12 (ii) ⇒ x = – 1 – 2y (iii) Here, x = 3 and y = – 2 Hence,

1 Topic The Substitution Method 2 Topic The Substitution Method California Standard 9 0 Students Solve A System Of Two Linear Equations Ppt Download

Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music Put this into the second equation 3 × (2 − y) 2y = 5 6 − 3y 2y = 5 6 − 5 = y y = 1 Since x y = 2 x = 2 −y = 2 − 1 = 1 Your solution x = 1 and y = 1Click here👆to get an answer to your question ️ Solve 3x 4y = 10 and 2x 2y = 2 by substitution method Solve Study Textbooks Join / Login >> Class 12 >> Applied Mathematics >> Linear Equations Solve by using substitution method 3 x 4 y = 1 0 & 2 x

 2xy = 3 Subtract 2x from each side 2x2xy = 32xy = 2x3 Multiple to each side by negitive one y = 2x3 > (2) From (2) substitute the y value in (1) 5x2(2x3) = 8 5x4x6 = 8 x6 = 8 Subtract 6 from each side x66 = 86 x = 2 Substitute the x value in (1) 5(2)2y = 8 102y = 8 Subtract 10 from each side y = 8102y = 2 Divide to eachSolve by Substitution 2x2y=3 , 2xy=5 2x 2y = −3 2 x 2 y = 3 , 2x − y = 5 2 x y = 5 Solve for y y in the second equation Tap for more steps Subtract 2 x 2 x from both sides of the equation − y = 5 − 2 x y = 5 2 x 2 x 2 y = − 3 2 x 2 y = 3Calculus questions and answers;

 Solve this system using the substitution method a – 4b = 2 5a = 3b – 7 Solve using the substitution method and be sure to show all of your work Substitution MethodPlz help Use the Substitution method to solve the system of equations x y = 4 x y = 2 solve for x in the second equation x= y2 Put that in for x in the first equation{y=2x14−4x−y=4 Enter your answer in the box Use the Substitution method to solve the system of equations y 2x = 5 3y x = 5 Solve one of the equations for x or y Let's solve the first one for y y 2x = 5 y = 2x 5 Now let's substitute 2x 5 for y in the second Solve the system of linear equations using the GaussJordan elimination method 3x−2y 4z = 22 2xy− 2z = 3 x

Solved 1 Solve By Graphing X 2y 6 X Y 3 2 Solve By Substitution Y 3 Course Hero

Using The Substitution Method To Solve A System Of Equations

{x−y=y=2x Enter your answer in the box 4What is the ycoordinate of the solution of the system of equations?Answer (1 of 5) 3x 9y = 3 (1) Divide both sides by 3, you get x 3y = 1 (1) 2x 6y = 2 (2) Divide both sides by 2, you get x 3y = 1 (2) You can see that (1) and (2) represent the same equation of a line, and for any real value of x, there is one and only one real value of y suUse the substitution method to solve the system of equations x 2y = 3 2x y = 24 Equation 1 is in the correct ax by format Equation 2 is in the correct ax by format Rearrange Equation 2 to solve for x 2x y = 24 Subtract y from both sides to isolate x 2x y y = 24 y 2x = 24 y This is our Revised Equation 2

Solve The Following Systems Of Equations X 2y 3 2 2x Y 3 2 Sarthaks Econnect Largest Online Education Community

Solved In Exercises 1 3 Solve The System By The Method Of Chegg Com

 Solve the system of linear equations using the GaussJordan elimination method 3x−2y 4z = 22 2xy− 2z = 3 x 4y − 8z = −16 Finite Math Solve the system of linear equations, using the GaussJordan elimination method Use the Substitution method to solve the system of equations x y = 4 x y = 2 solve for x in the second The system of equations are Write the equations into matrix form is coefficient matrix, is variable matrix and is constant matrix Solve the equations in Gaussjor dian method The augmented matrix is , R3 are represents first row, second and solve by substitution method;x2y=3/2;

How Do You Solve The System 2 X 4 Y 6 3x 2 Y 3 13 Socratic

2 2 Y 3 Store 53 Off Www Nogracias Org

By Gausslordan Method, solve the following system of linear equations xyz w = 10 x yzw = 2 2x 2z = 8 2y Zw = 12 x 3y z3w = 22Y^2 = x^32x^2 Natural Language;X 2(3) = 4 x – 6=4 x = 46 x = 2 Therefore, the value of x is 2 Hence, the solution for the system of linear equations is x = 2 and y=3 To check whether the obtained solution is correct or not, substitute the values of x and y in any of the given equations Verification Use Equation (2) to verify the solution x2y = 4 Now

How Do You Solve The System 2 X 4 Y 6 3x 2 Y 3 13 Socratic

Systems Of Linear Equations

Question Solve the system using the substitution method x 2y = 6 3x 2y = 2 This is what I did 3x 2y = 2 x = 2y 3 _____ (2y 3) 2y = 6 = 2y 2y = 3 _____ y= 3 _____ x = 2y 3 x= 2 (3) 3 x = 9 I checked my solution and it was wrong I don't know where I messed up Answer by jerryguo41(197) (Show Source) Using substitution method 3x2 (x6)=8 3x2x12=8 5x= x=4 Now substitute x in one of the two equations given to find y y=46 y=2 The solution is (4,2)Solve by Substitution y=x3 , y=2x4 y = x 3 y = x 3 , y = 2x 4 y = 2 x 4 Eliminate the equal sides of each equation and combine x3 = 2x4 x 3 = 2 x 4 Solve x3 = 2x4 x 3 = 2 x 4 for x x Tap for more steps Move all terms containing x x to the left side of the equation

If X Y 3 X Y 1 Then What Is X Y Quora

1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 3 5 3x 2y 61 60 Respected Teachers Please Find The Value Of X And Y Mathematics Topperlearning Com 5qbsqgee

 Substitute (3) into either of (1) or (2) to find the value of y color(blue)("Method 2") 5/2x3/2 =y =2x6 5/2x3/2 =2x6 Now solve for x and substitute in (1) or (2) to find y color(red)("Both methods are fast once you are") color(red)("able to "underline("change the variable by sight")Textbook Solutions Expert Tutors Earn Main Menu;Substitution method x2y=2x5, xy=3 en Related Symbolab blog posts High School Math Solutions – Systems of Equations Calculator, Elimination A system of equations is a collection of two or more equations with the same set of variables In this blog post,

Systems Of Equations With Substitution 2y X 7 X Y 4 Video Khan Academy

Solve By Substitution Method 3x 5y 1 And 2x Y 3 Scholr

3 x 4 y = 1 0 —(1) 2 x − 2 y = 2 By substitution method, 2 x − 2 y = 2 ⇒ 2 (x − y) = 2 ⇒ x − y = 1 ∴ x = y 1 → (2) Substituting x = y 1 in (1) we get ∴ 3 x 4 y = 1 0 ⇒ 3 (y 1) 4 y = 1 0 ⇒ 3 y 3 4 y = 1 0 ⇒ 7 y = 7 ⇒ y = 1 ∴ x = 1 1 = 2 Answer x = 2, y = 1Substitute \frac {2y} {3} for x in the other equation, 2xy=3 Substitute 3 2 y for x in the other equation, 2 x − y = 3 2\left (\frac {1} {3}y\frac {2} {3}\right)y=3 2 ( 3 1 y 3 2 ) − y = 3 Multiply 2 times \frac {2y} {3} Multiply 2 times 3 2 y \frac {2} {3}y\frac {4} {3}y=3Question 2 Substitution method xy=16 and y=3x 3 Solve the system by the

Can You Draw X 2 Y 2 1 3 X 2y 3 Quora

3 2 Substitution Method Notebook

Solve 2xy/ X Y = 3/2 Xy/ 2x Y = 3/10 X Y ≠ 0 and 2x Y ≠ 0 CISCE ICSE Class 9 Question Papers 10 Textbook Solutions Important Solutions 15 Question Bank Solutions Concept Notes & Videos 430 Syllabus Advertisement Remove all ads Solve 2xy/ X Y = 3/2 Xy/ 2x Y = 3/10 X Y ≠ 0 and 2x Y ≠ 0Simultaneousequationscalculator substitution method x2y=2x5, xy=3 en Related Symbolab blog posts Middle School Math Solutions – Simultaneous Equations Calculator Solving simultaneous equations is one small algebra step further on from simple equations 2x 3y = 3 and x 2y =2 Recent Visits Q&A;

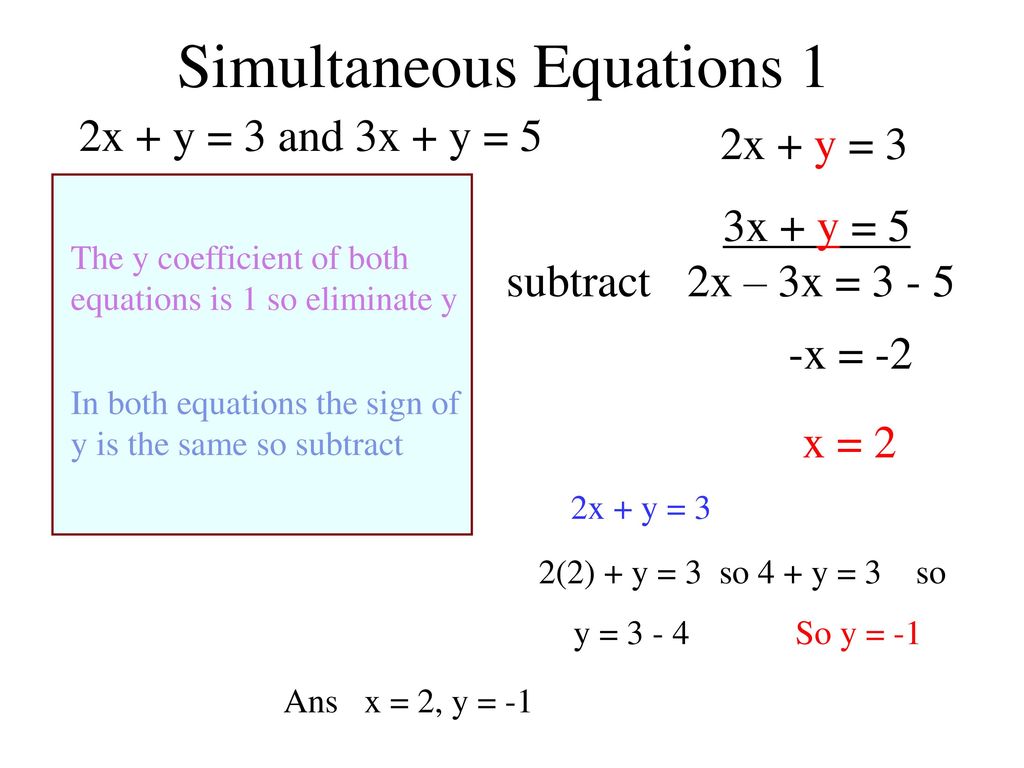
Simultaneous Equations 1 Ppt Download

Solve The Following Pairs Of Linear Equations By The Substitution Method 3x 2 5y 3 2 X 3 Y 2 13 6 Sarthaks Econnect Largest Online Education Community

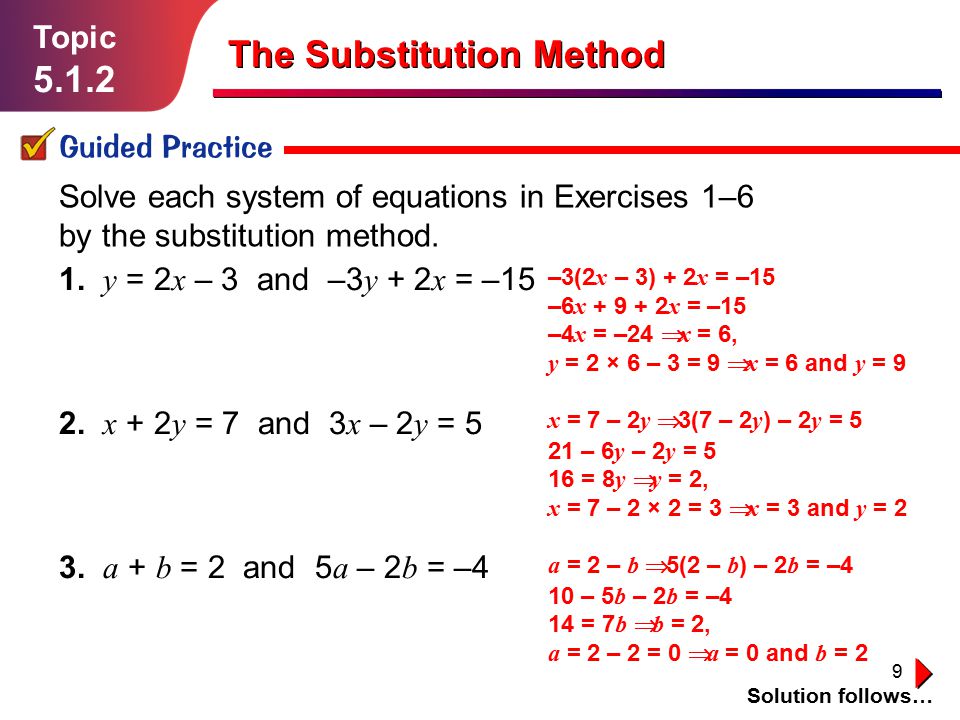
{y=2x−64x−2y=14 Use the substitution method 2Solve {y=x−63x2y=8 Use the substitution method 3What is the ycoordinate of the solution for the system of equations?Substitution method x2y=2x5, xy=3 \square!Q2 4x 2y = 16 and 2x y = 11 (substitution method) 2x y = 11 y = 11 2x Use y = 11 2x 4x 2(112x) = 16 4x 22 – 4x =16 x = 16 22 x = 6 Use X = 6 2(6) y = 11 12 y = 11 y = 11 – 12 y= 1 Answer x = 6 and y = 1 Q3 2/3 x ¼ y = 3/2 and ½ x – ¼ y = 2 (substitution method) Simplify 2 x 1 y = 3 = 8x 3y = 18

Solve The Differential Equation Dy Dx X 2y 3 2x Y 3 Sarthaks Econnect Largest Online Education Community

Solved Exercises 5 18 Solve Each System By The Substitution Method Fx Y 4 6 Jx Y 6 Ly 3x Y 2x Fx Jy 8 J2x 8 3y

 Answerx=7 and y=10Stepbystep explanationy=x3 eqn (1)y=2x4 eqn(2)From equation 1x=y3 eqn (3)Subtitude equation 3 into equation 2y=2( nonone nonone Mathematics High School answered Solve the following system of equations by using the substitution method y = x 3 and y = 2x – 4 2 See answers Advertisement`3xy=3` and `7x2y=` `2xy=11` and `5x4y=1` `2x7y=1` and `4x3y=15` `3x5y=1` and `5x2y=19` `5x8y=9` and `3y2x=4` Share this solution or page with your friends Solve linear equation 7y2x11=0 and 3xy5=0 using Substitution Method, stepbystep online We use cookies to improve your experience on our site and to show you relevant Click here 👆 to get an answer to your question ️ xy/2=4 and x/32y=5 by substitution method komal2131 komal2131 Math Secondary School answered Xy/2=4 and x/32y=5 by substitution method 2 2x=6 x=3 hence ,x=3 and y=2 New questions in Math chL be bandar nikhal shut up bs shut up kar diya na dek liyoo

X 2 2y 3 1 And X Y 3 3 Solve By Elimination Method Brainly In

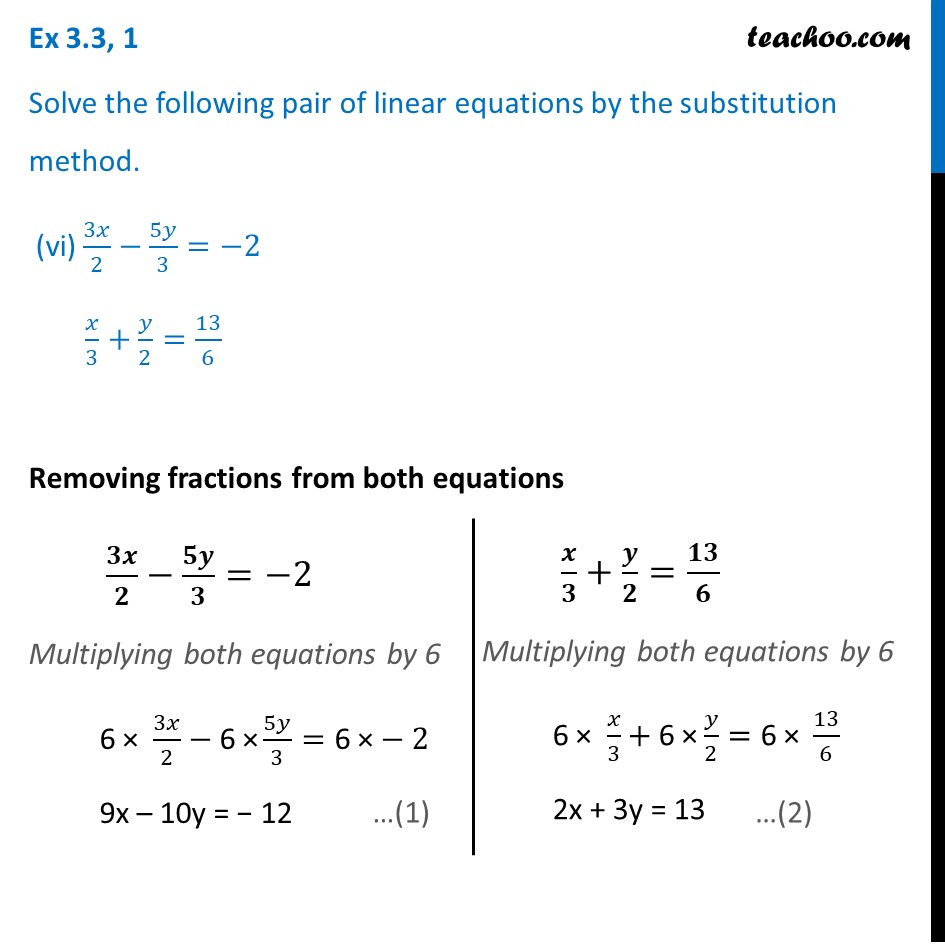
Solve The Pair Of Linear Equations By Substitution 3x 2 5y 3 2

X2y=6 6y=x18 a 18 b 0*** c 6 d 3 2 Solve the following system of equations using substitution What is the value of y? We make y the subject in equation (2) to get, We put equation (3) into equation (1) to obtain, We group like terms to get, This implies that, We divide through by to get, Hence the xcoordinate is QUESTION 3 The given system is and We make the subject in equation (2) to get, We put equation (3) into equation (1) to obtain, We expand the Radio signals travel at a rate of 3 × 108 meters per second How many seconds will it take for a radio signal to travel from a satellite to the surfac e of the Earth if the satellite is orbiting at a height of 75 × 106 meters?

Solve Dy Dx 2x Y 1 X 2y 3 Youtube

How To Solve This Differential Problem Dy Dx X 2y 3 2x Y 3 Quora

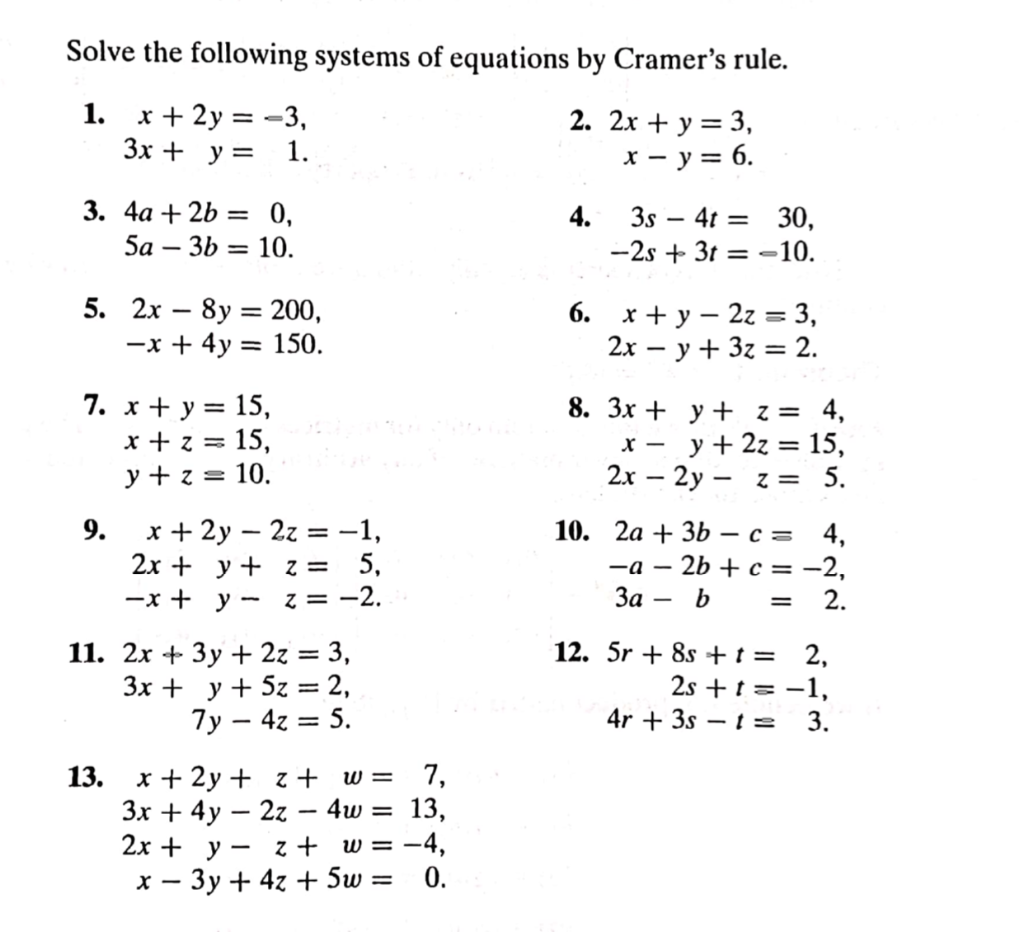
M5 – Elimination to Gauss – Jordan Method 1) x 2y z = 3 2) 2x – y 2z = 6 3) 3x y – 2 = 5 Ax ROW 1 ROW 2 ROW Study Resources Main Menu;Welcome to Mathskeycom solve this system of equations by using the substitution method 5x3y=11 (1) and 3x22y=1 (2) asked in ALGEBRA 2 by mathgirl Apprentice systemofequations; 1 For the following system of equations, what is the xvalue of the solution?

If 2x 2y 7 And 3x 2y 3 Then X

Solve X 2y 2 2x Y 16

Let us take an example of solving two equations x2y=8 and xy=5 using the substitution method Let us assume two linear equations 2x3(y5)=0 and x4y2=0 Step 1 Simplify the given equation by expanding the parenthesis if needed So, here we can simplify the first equation to get 2x 3y 15 = 0 Now we have two equations as,2x3y=105 x2y=65 a Algebra PLEASE HELP!!The normal to the ellipse at the point (x,y,z) is \nabla(x^2y^24z^2) = (2x, 2y, 8z) At minimum or maximum distance to the plane, More Items Share Copy Copied to clipboard x\left(x^{2}2xyy^{2}\right) Factor out x \left(xy\right)^{2} Consider x^{2}2xyy^{2} Use the perfect square formula, a^{2}2abb^{2}=\left(ab\right)^{2

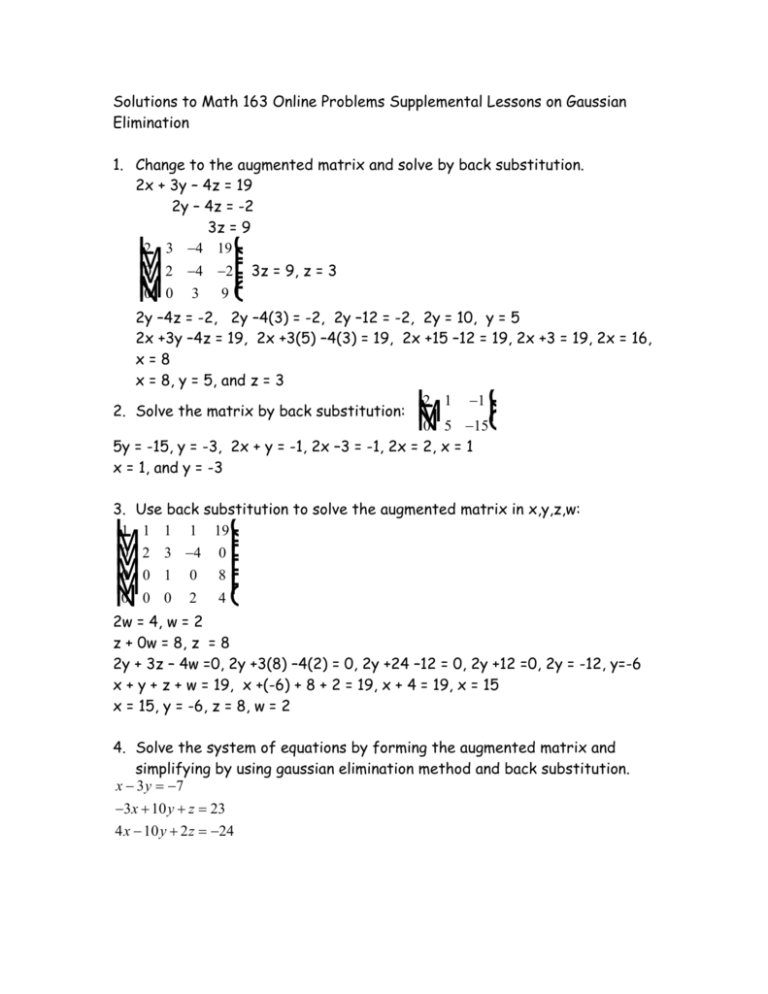
Math 163 Online Problems Supplemental Lessons On Gaussian

How To Solve Simultaneous Equations Using Substitution Method

 Transcript Example 7 Solve the following pair of equations by substitution method 7x – 15y = 2 x 2y = 3 7x – 15y = 2 x 2y = 3 From (1) 7x – 15y = 2 7x = 2 15y x = (𝟐 𝟏𝟓𝒚)/𝟕 Substituting the value of x in (2) x 2y = 3 (2 15𝑦)/7 2𝑦=3 Multiplying both sides by 7 7 × ((2 15𝑦)/7) 7×2𝑦=7×3 (2 15y) 14y = 21 15y 14y = 21 – 2 29y = 21 – 2Are solved by group of students and teacher of Class 10, which is also the largest studentIs done on EduRev Study Group by Class 10 Students The Questions and Answers of Solve using substitution method 3x/25y/3=2 , x/2 y/2=13/6?ans x=2,y=3?

X 2 2y 3 1 X Y 3 3 Solve The Given Equation Using Elimination And Substitution Method Youtube

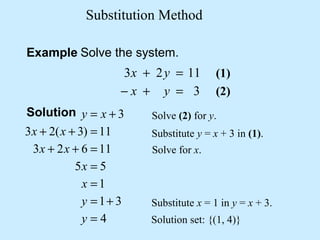
Solving Systems Of Linear Equations

Solve by Substitution 3xy=2 , x2y=3, Subtract from both sides of the equation Replace all occurrences of in with Simplify Tap for more steps Simplify each term Tap for more steps Apply the distributive property Multiply by Multiply bySolve the system by the substitution method y= 2x 7 and 2x 3y = 19 4 xy= 1 and xy= 5 Solve the system by the addition method 5 Solve the system by the addition method 4x13y= 8 and 2x13y=4 6 Solve the system by graphing 3x2y=12 and x 2y= 4;What advantages does the method of substitution have over graphing for solving systems of

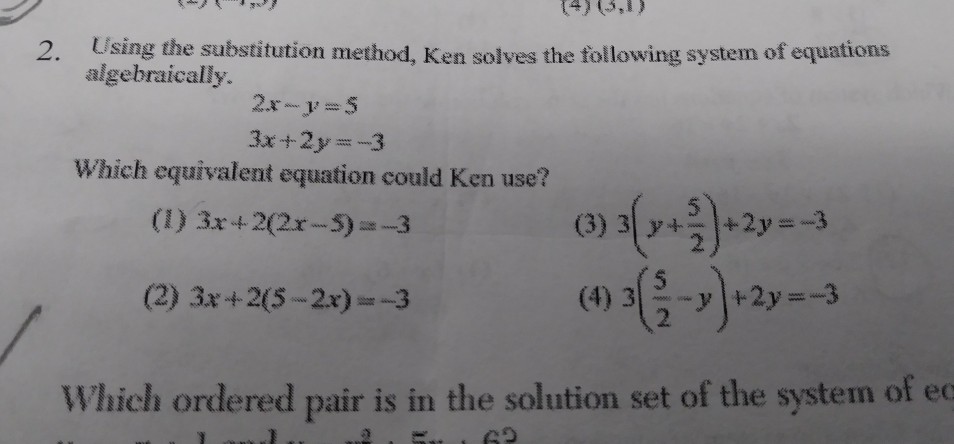
Solved 43 1 Using The Substitution Method Ken Solves The Chegg Com

2x 3y 8 X 2y 3 0 By Substitution Method Brainly In

2xy=3/2 Brainlyin Click here 👆 to get an answer to your question ️ solve by substitution method;x2y=3/2;Answer to Using Laplace transforms, find the solution for the initial value problem \\\\ \\dfrac{d^2y}{dx^2} 3 \\dfrac{dy}{dx} 2y = 4e^{2x}\\6 seconds 25 × 10–2 seconds 40

1 Topic The Substitution Method 2 Topic The Substitution Method California Standard 9 0 Students Solve A System Of Two Linear Equations Ppt Download

Solve 1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 1 5 3x 2y 61 60 Mathematics Topperlearning Com 56o23roo

Earn Free Access Jordan Method 1) x 2y z = 3 2) 2x – y 2z = 6 3) 3x y – 2 = 5 Ax B This discussion on Solve using substitution method 3x/25y/3=2 , x/2 y/2=13/6?ans x=2,y=3?  2x −y = 3 x 2y = 24 Substitution is a method for solving algebraic equations by finding the value of a variable in terms of other variables and substituting that value into existing equations x = 24 − 2y Substituting 24 −2y for x in the first equation 2(24− 2y) −y = 3 48 −4y − y = 3 5y = 48 −3

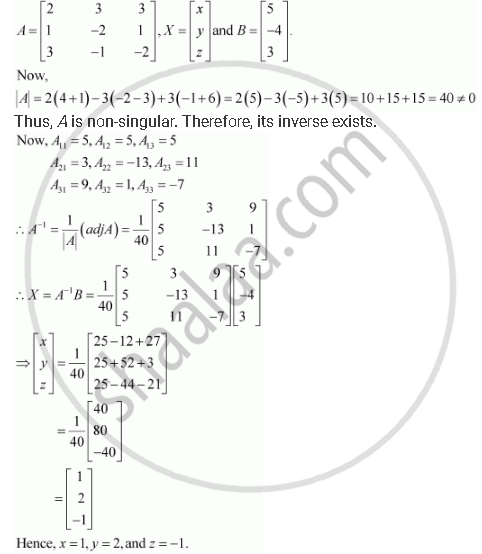
4 Solve The Following System Of Linear Equations Using The Inverse Matrix Method 1 Y Homeworklib

1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 3 5 3x 2y 61 60 Respected Teachers Please Find The Value Of X And Y Mathematics Topperlearning Com 5qbsqgee

Algebra Solve by Substitution y=2x3 , y=x3 y = 2x − 3 y = 2 x 3 , y = −x 3 y = x 3 Eliminate the equal sides of each equation and combine 2x−3 = −x3 2 x 3 = x 3 Solve 2x−3 = −x3 2 x 3 = x 3 for x x Tap for more steps Move all terms containing xTranscribed image text SUBSTITUTION METHOD WORKSHEET HOMEWORK OUIZ 1) 2x 8y 2) x=5 2x y=10 3) 5x 2y 3 y=2x 4) 2y x=15 x=3y 5) 4x 7y 19 y=x9 6) y 6x 11 2y4x = 14 Previous question Next question Get more help from Chegg Solve it with our algebra problem solver and calculator

2 Y 3 Y 4 Cheap Sale 56 Off Www Nogracias Org

X 2y 3 2 2x Y 3 2 Solve This Equation Using Substitution Method Brainly In

Solve The Pair Of Linear Equations By Substitution 3x 2 5y 3 2

October 12 Mathpowerblog

The Substitution Method

Solve The Following Simultaneous Linear Equation By Substitution Method 2x 3y 4 3 5x 2y 7 0 Brainly In

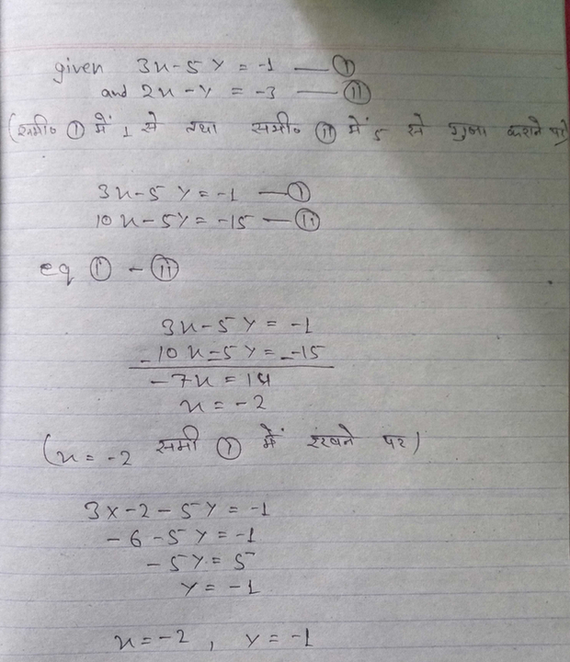
Solve By Substitution Method 3x 5y 1 And 2x Y 3 Scholr

Solved Help Me Check My Work On Questions 3 9 13 Only Chegg Com

J19

Search Q X 2 2b2y 3 3d 1 And X Y 3 3d3 By Substitution Method Tbm Isch

7x 15y 2 X 2y 3 Solve Using Substitution Method Youtube

How To Solve Using The Gauss Elimination Method Y Z 2 2x 3z 5 X Y Z 3 Quora

Example 7 Solve By Substitution 7x 15y 2 X 2y 3

Let S Learn Solving Systems Using The Substitution Method

9 Quant 2 Equations And 2 Variables Flashcards Quizlet

Solve The Following Pair Of Equations By Substitution Method X Y 1 And 2x 3y 2

Solve By Substitution Method 2x Y 3 0 4x Y 5 0 Maths Pair Of Linear Equations In Two Variables Meritnation Com

3 2 Y 1 Shop 57 Off Www Nogracias Org

Answered 2 Using The Substitution Method Ken Bartleby

Solve The Following Systems Of Equations X 2y 3 2 2x Y 3 2 Brainly In

X 7 Y 3 5 X 2 Y 9 6

X 2y 3 2 And 2x Y 3 2 By Elimination Method Brainly In

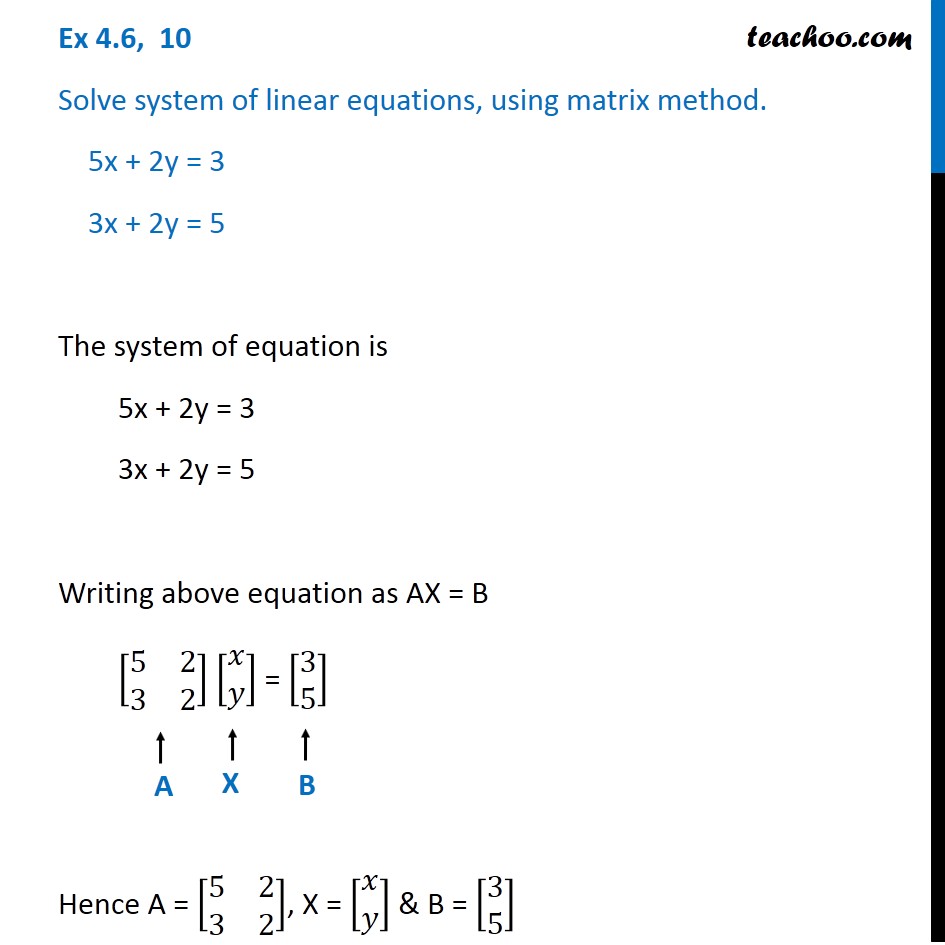
Ex 4 6 10 Solve Using Matrix Method 5x 2y 3 3x 2y 5 Ex 4 6

The Substitution Method

Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 3

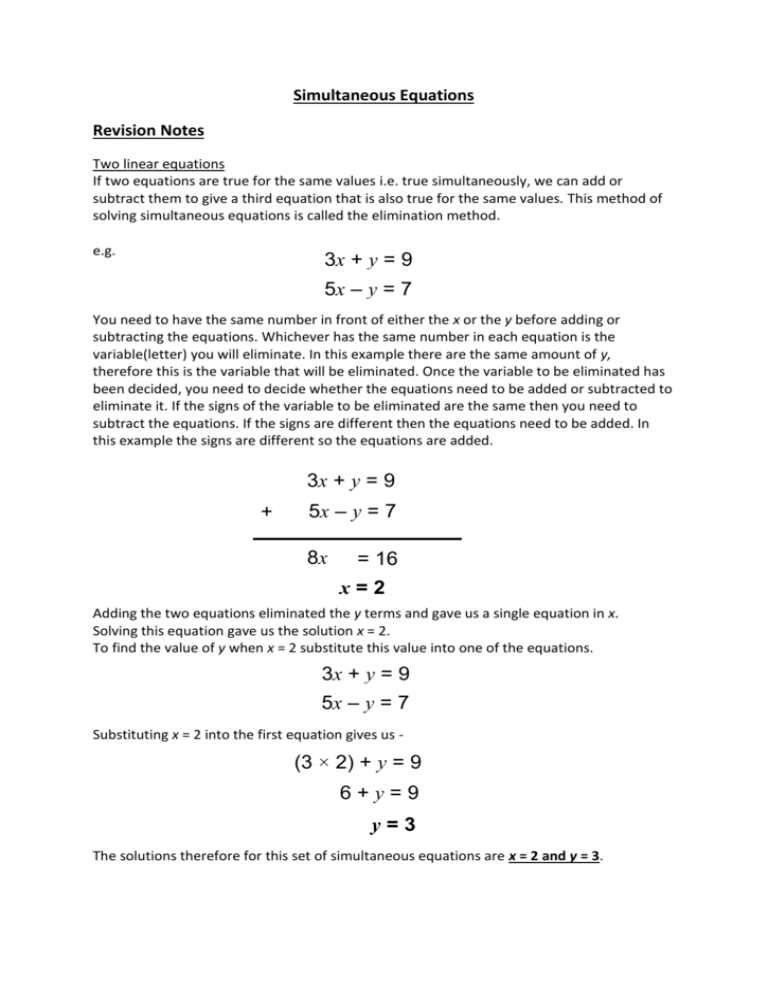
Simultaneous Equations

3k 4r 2m 2 3k 4r 2m 2

Solved I Need To Find The Solution Of These 4 Questions Using Substitution I M Super Stuck Tc Suiution Is 2 1 Check Substitute Your Solution Into Either Ofthe Given Linear Equations X 3

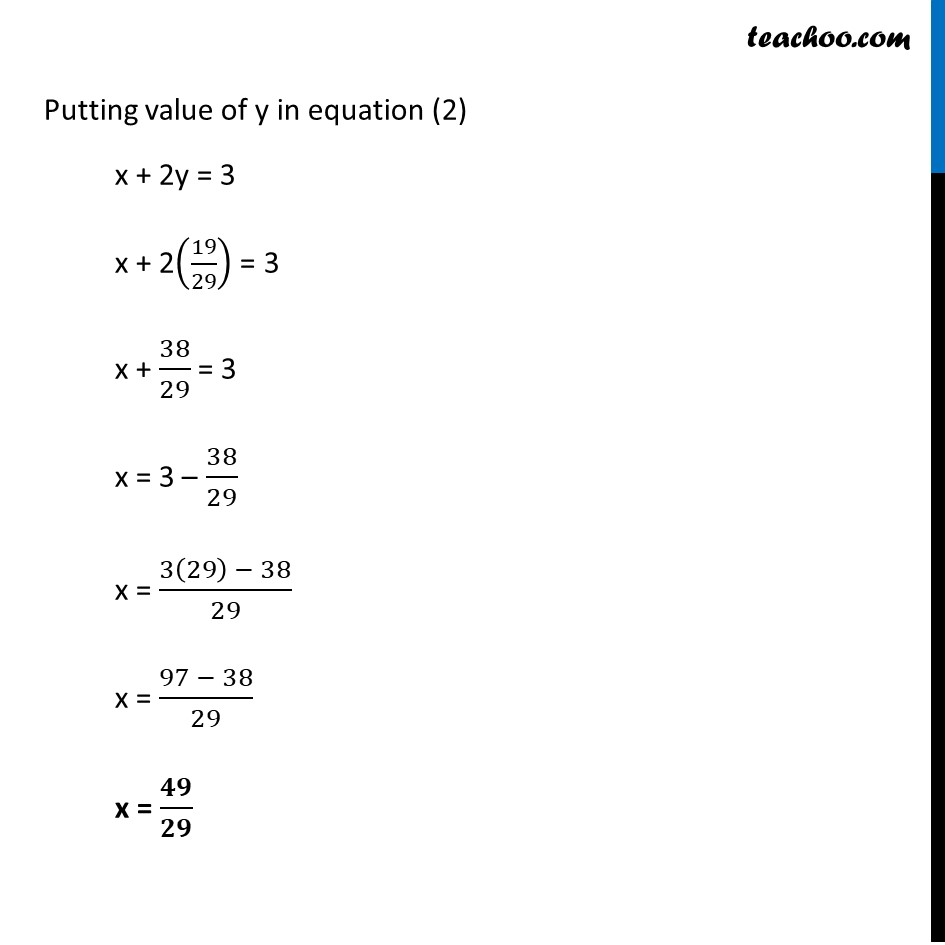
Example 7 Solve By Substitution 7x 15y 2 X 2y 3

Solved Solve The System Of Equations Y 2x 3 And 2y 2x 8 Using The Course Hero

2 3 Y 5 Cheap Sale Up To 55 Off Www Editorialelpirata Com

3 Systems Of Linear Equations

Solved Solve The System Of Equations Y 2x 3 And 2y 2x 8 Using The Course Hero

Solve The Following Systems Of Equations 1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 3 5 3x 2y 61 60 Sarthaks Econnect Largest Online Education Community

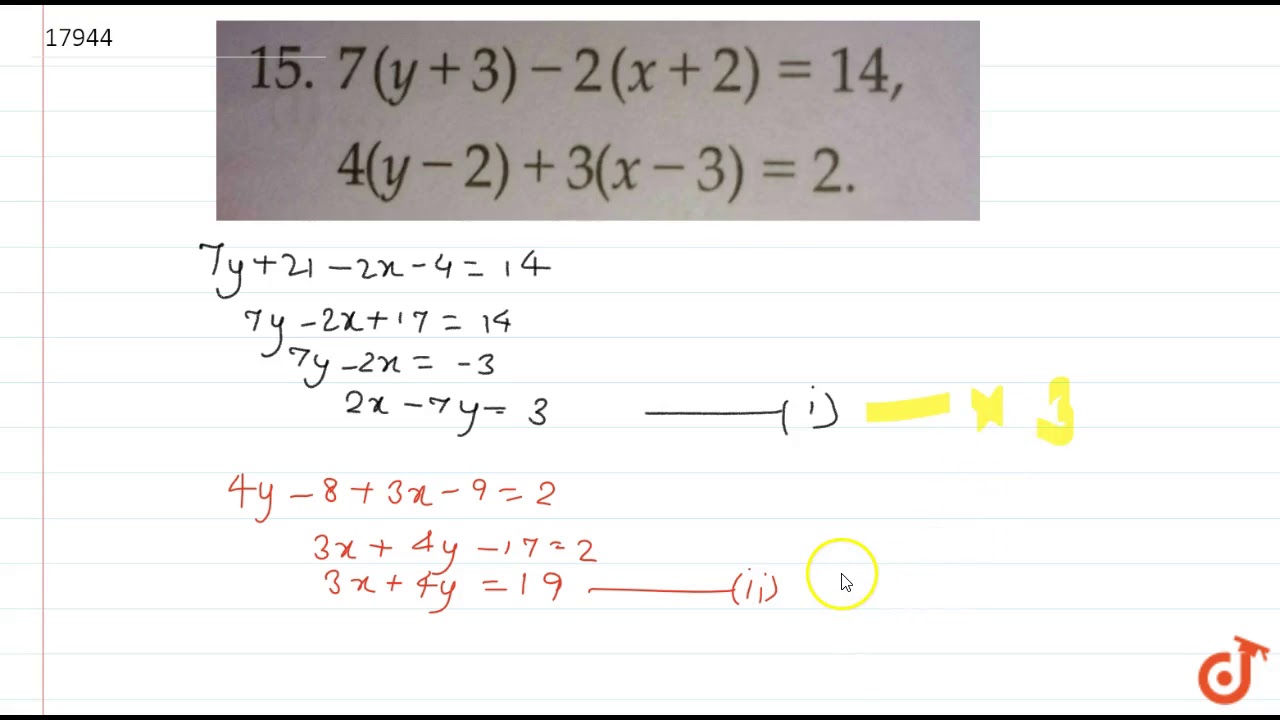
7 Y 3 2 X 2 14 4 Y 2 3 X 3 2 Youtube

X 2y 3 2 2x Y 3 2

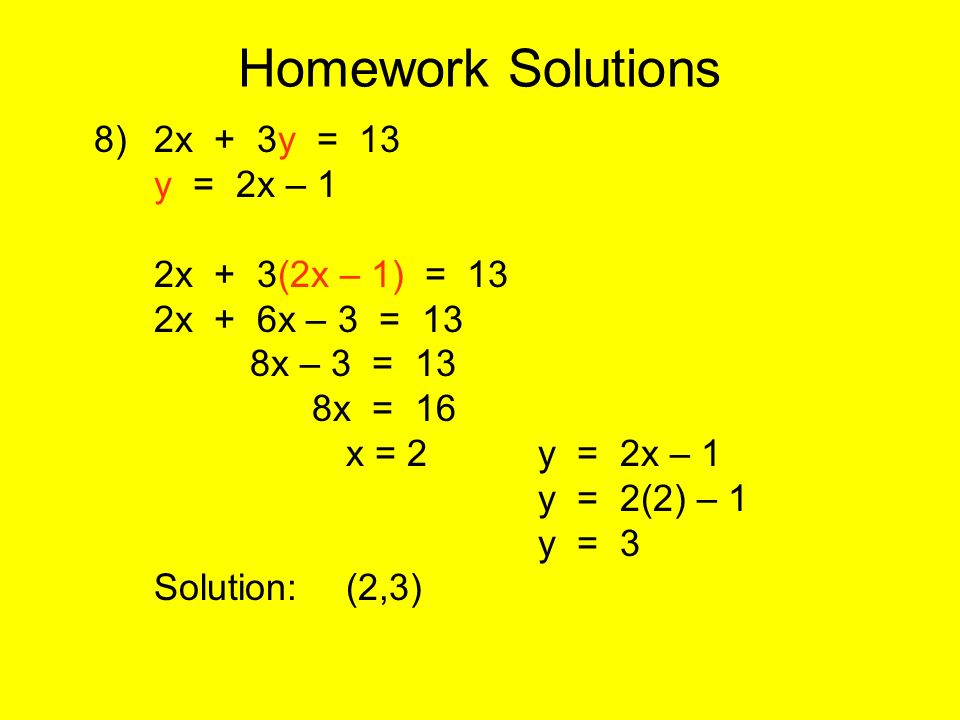
3x 5y 11 X 3y 1 Do Now Homework Solutions 2 2x 2y 6 Y 2x 2x 2 2x 6 2x 4x 6 6x 6 X 1y 2x Y 2 1 Y Ppt Download

Solve The Following Pairs Of Linear Equations By The Elimination Method And The Substitution Method Ii X 2 2y 3 1 And X Y 3 3 Sarthaks Econnect Largest Online Education Community

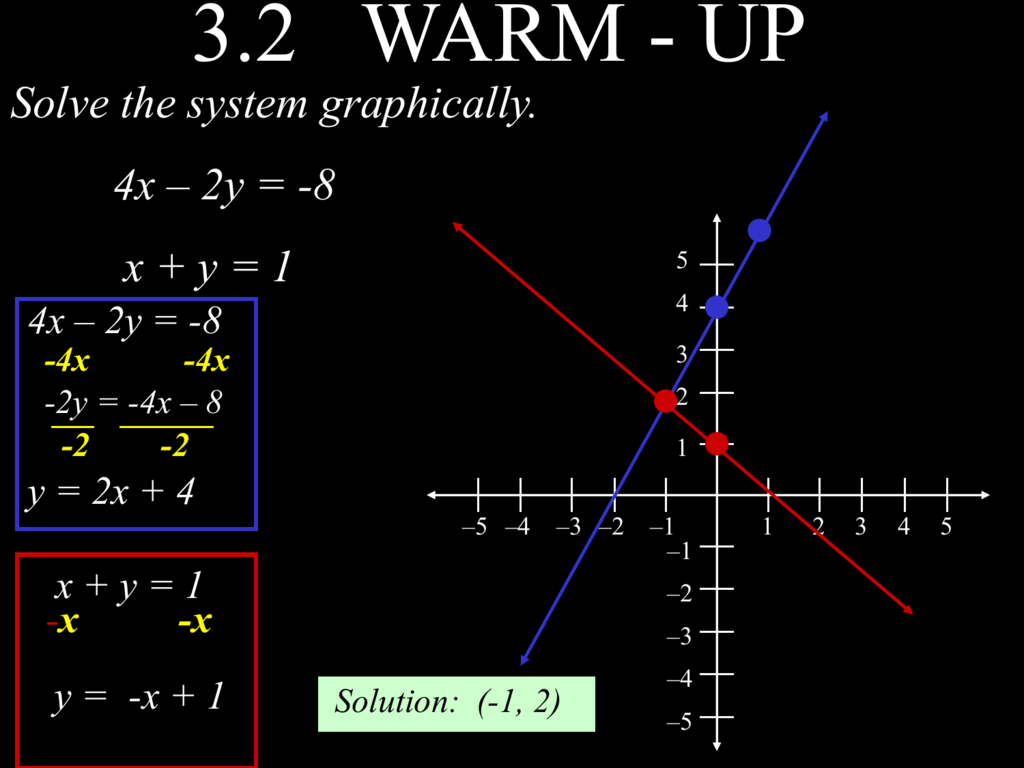
Ch 3 2

What Is The General Solution Of The Differential Equation 2x 2y 3 Dy Dx X Y 1 Quora

How To Solve The Following Simultaneous Equations By Elimination Method A 2x 3y 12 X Y 1 B 3x Y 10 C X 3y 1 3x 2y 4 0 D 5 Quora

Solve The Following Systems Of Equations 1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 3 5 3x 2y 61 60 Sarthaks Econnect Largest Online Education Community

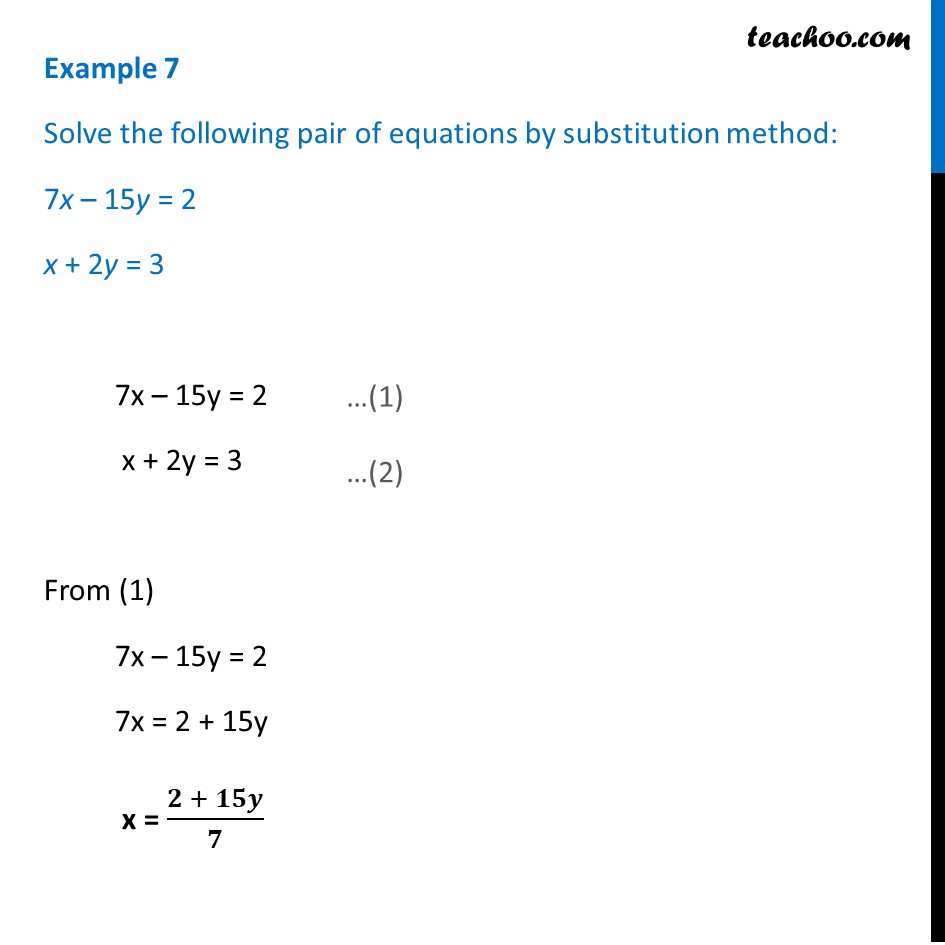
Example 7 Solve By Substitution 7x 15y 2 X 2y 3

Solve The Following Pair Of Linear Equations By The Substitution Method 0 2 X 0 3y 1 3 0 4x 0 5y 2 3

Solved I Solve By Making A Substitution To Reduce The Given Chegg Com

X 2y 3 2 2x Y 3 2 Solve This Equation Using Substitution Method Brainly In

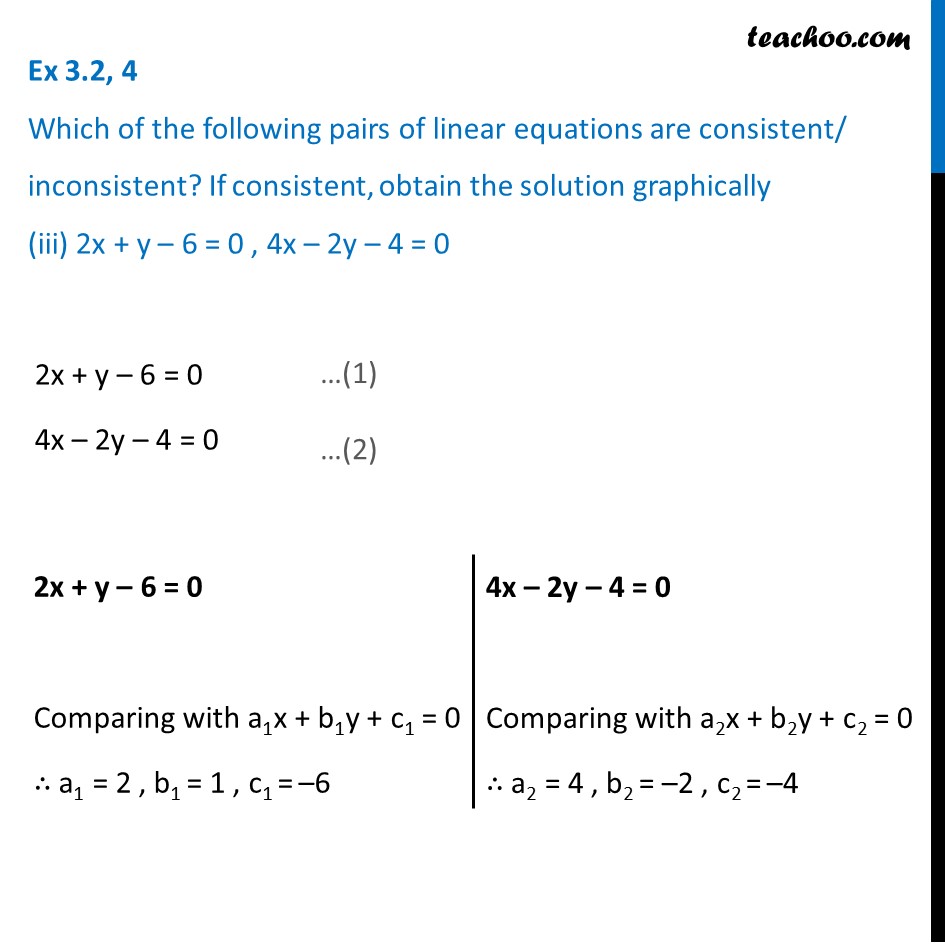
Ex 3 2 4 Iii Check If Equations 2x Y 6 0 4x 2y 4 0

Answered Solve The Following Systems Of Bartleby

How Do You Solve X 2y Z 2 2x 3y 2z 2 And 4x 8y 5z 5 Using Matrices Socratic

3 Systems Of Linear Equations

7 Y 3 Clearance Sale Up To 53 Off Www Editorialelpirata Com

Series 575 552 533 518 507 500 A Find The Value Of A

Ordinary Differential Equations Solve 2x 3 2 Frac D 2y Dx 2 2x 3 Frac Dy Dx 12y 6x Mathematics Stack Exchange

Solve The Following Simultaneous Equations By The Method Of Equating Coefficients 2x Y 3 3x 2y 3 4x 3y 5x 4y 4 1

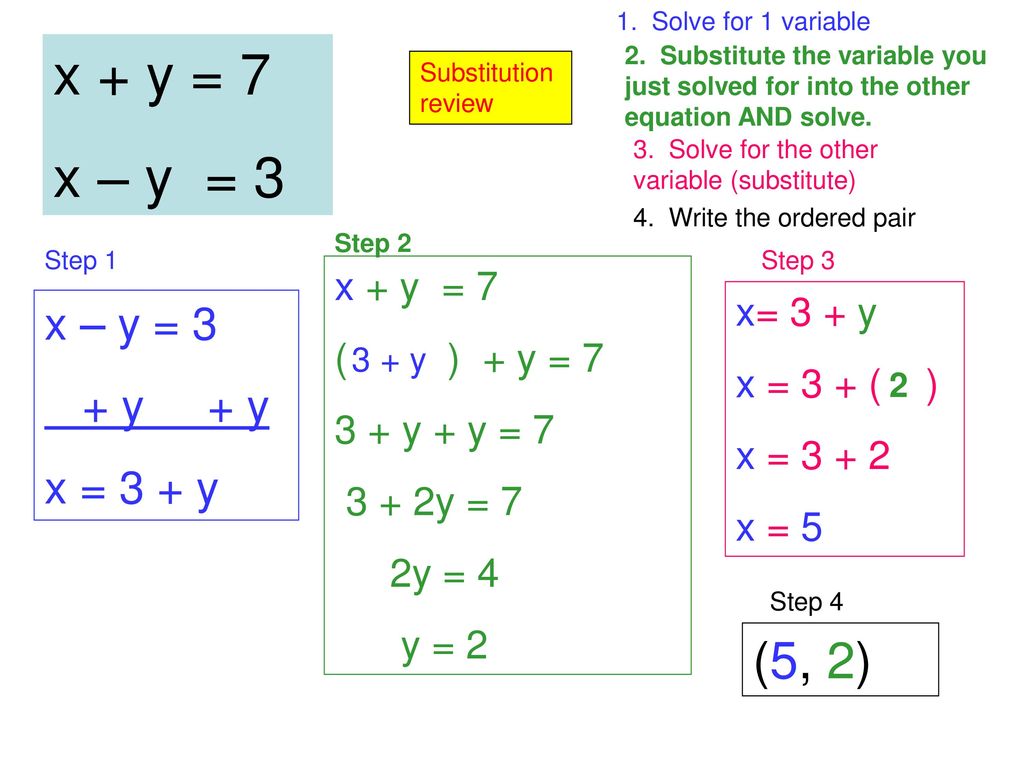
Elimination Method Day 1 Ppt Download

How To Solve Simultaneous Equations Using Substitution Method

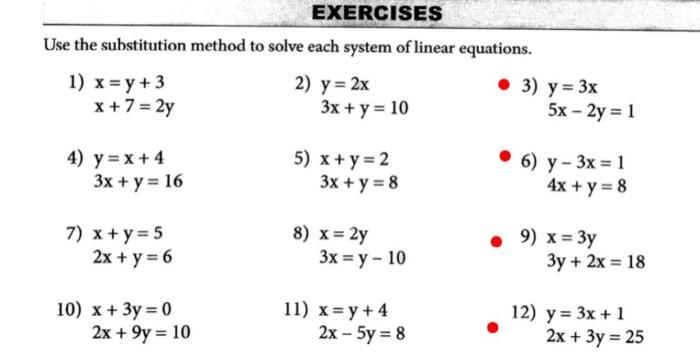
Solved Exercises Use The Substitution Method To Solve Each Chegg Com

Tre 1 Solve The Following Pairs Of Equations By Reducing Th Scholr

Solved Solve By Using Substitution Method 2x 3y 2 X Chegg Com

2 Y 3 Y 4 Cheap Sale 56 Off Www Nogracias Org

Solve The Pair Of The Following Equation By Substitution Method A 3x 2 5y 3 2 X 3 Y 2 13 6 Maths Pair Of Linear Equations In Two Variables Meritnation Com

Solve By Matrix Method 2x 3y 3z 5 X 2y Z 4 3x Y 2z 3

Graph Graph Inequalities With Step By Step Math Problem Solver

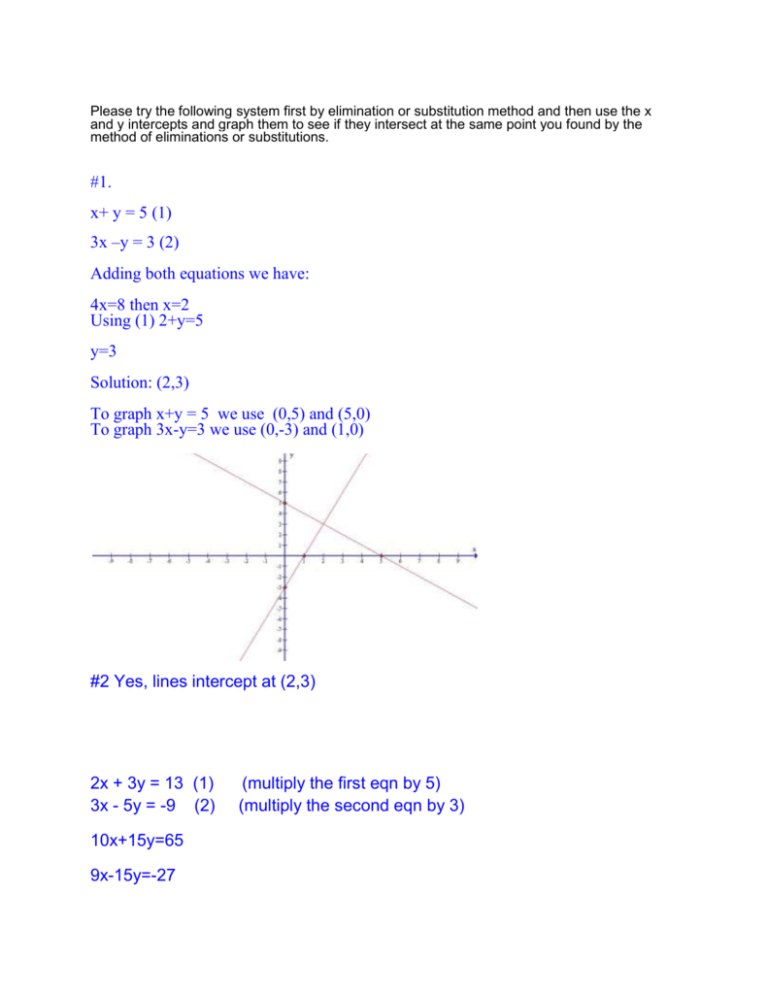
3x Y 3 Justanswer

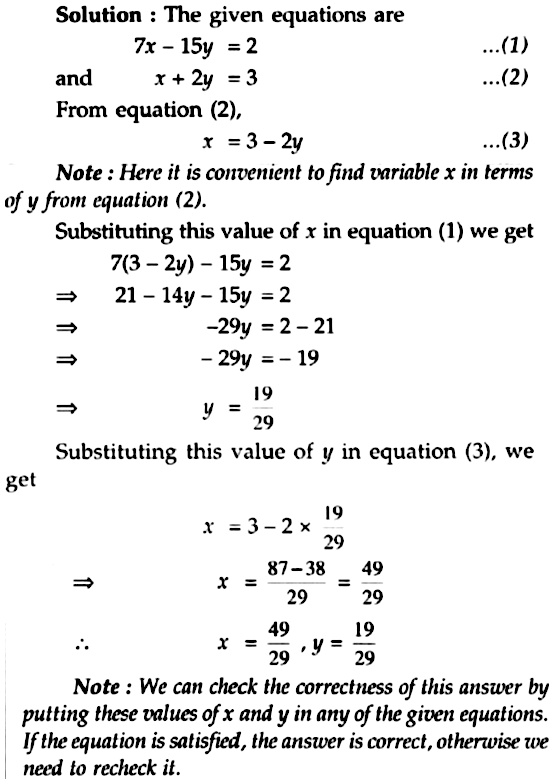
Example 1 Solve The Following Pair Of Equations Bysubstitution Method 7x 15y 2x 2y 3 Snapsolve

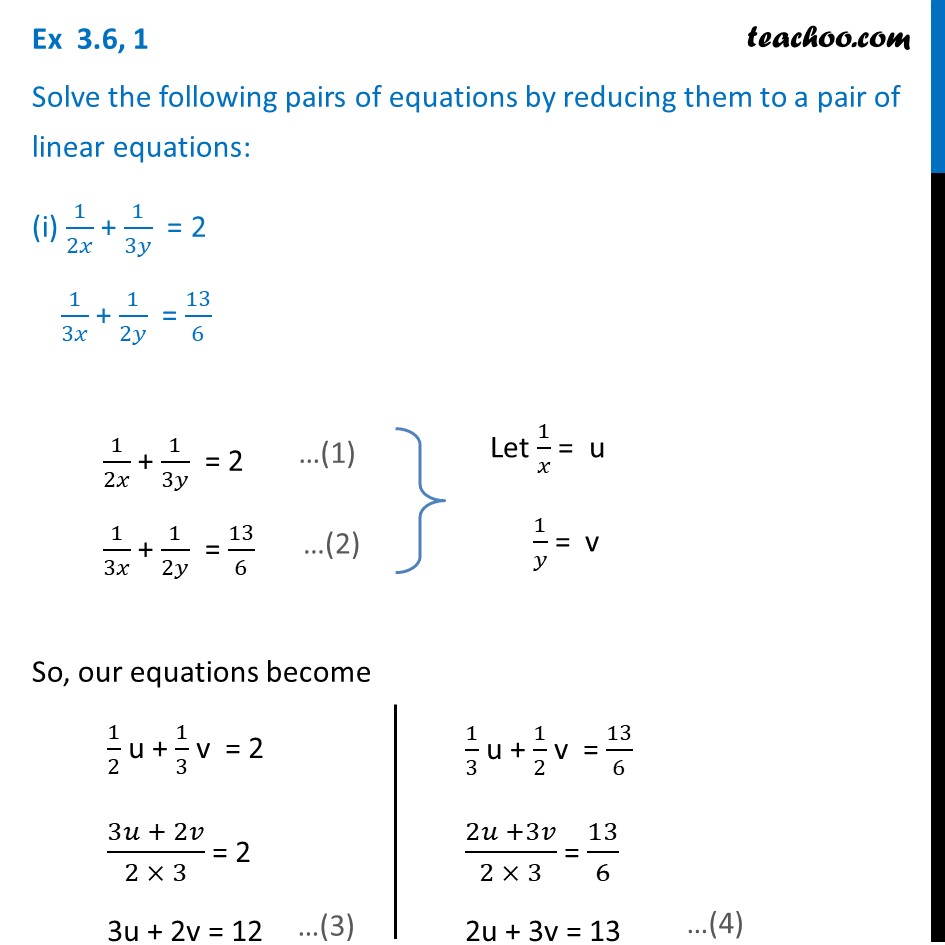
3 2 Y 1 Shop 57 Off Www Nogracias Org

X 2 2y 3 1 And X Y 3 3 Find X And Y Values Using Elimination And Substitution Method Youtube

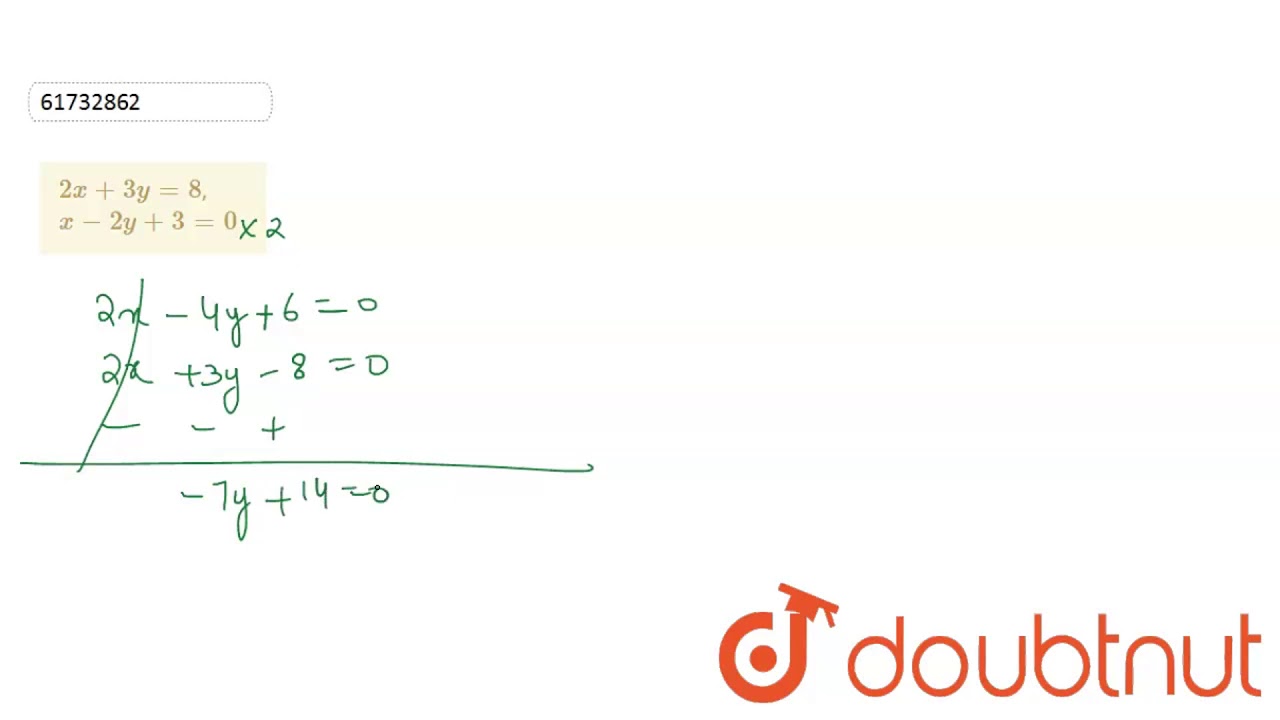
2x 3y 8 X 2y 3 0 Youtube

7 Y 3 2 X 2 14 4 Y 2 3 X 3 2 Mathematics Topperlearning Com Yy1pzkhh

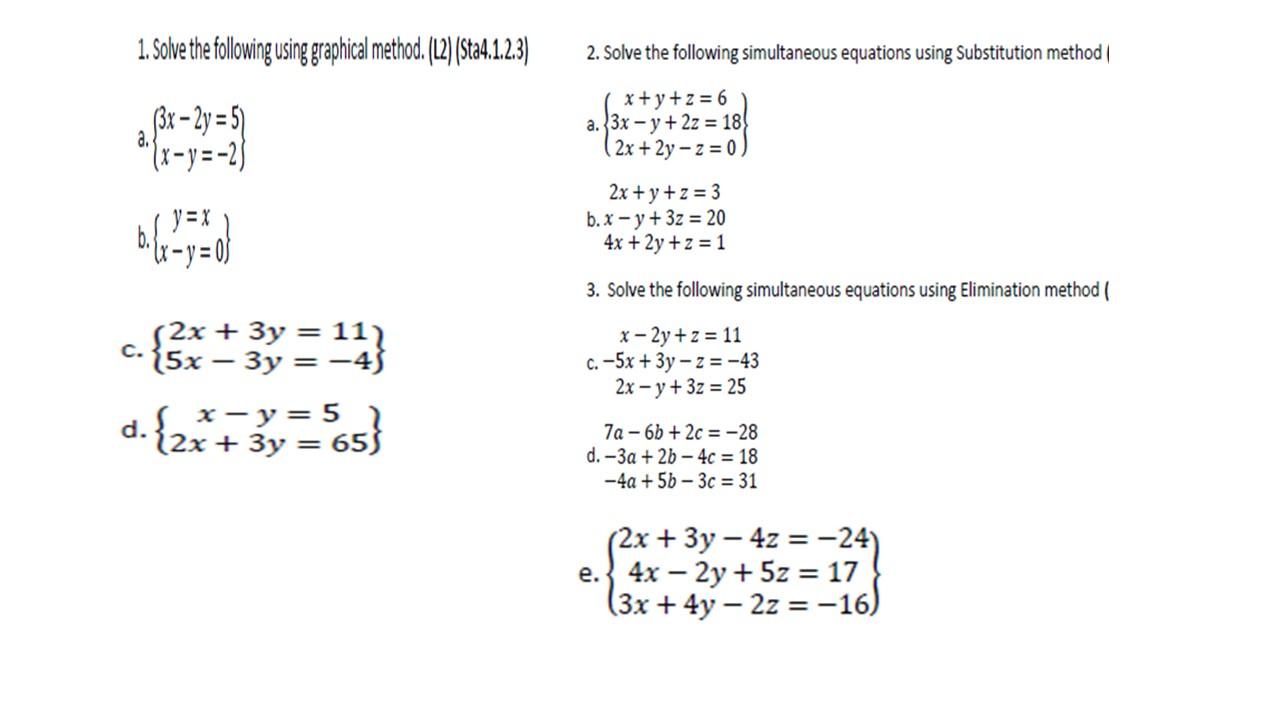
Solved 1 Solve The Following Using Graphical Method 12 Chegg Com

Incoming Term: x+2y=3/2 2x+y=3/2 by substitution method,